Quantum Spin Hall Effect

The quantum spin Hall state is a state of matter proposed to exist in special, two-dimensional, semiconductors with spin-orbit coupling. The quantum spin Hall state of matter is the cousin of the integer quantum Hall state, but, unlike the latter, it does not require the application of a large magnetic field. The quantum spin Hall state does not break any discrete symmetries (such as time-reversal or parity). It has been recently proposed and subsequently experimentally realized in mercury (II) telluride (HgTe) semiconductors.

The first proposal for the existence of a quantum spin Hall state was developed by Kane and Mele who adapted an earlier model for graphene by Haldane which exhibits an integer quantum Hall effect. The Kane and Mele model is two copies of the Haldane model such that the spin up electron exhibits a chiral integer quantum Hall Effect while the spin down electron exhibits and anti-chiral integer quantum Hall effect. Overall this idealized model has a charge-Hall conductance of exactly zero but a spin-Hall conductance of exactly:

(in units of ).

Independently, a quantum spin Hall model was proposed by Bernevig and Zhang in an intricate strain architecture which engineers, due to spin-orbit coupling, a magnetic field pointing upwards for spin-up electrons and a magnetic field pointing downwards for spin-down electrons. The main ingredient is the existence of spin-orbit coupling, which can be understood as a momentum-dependent magnetic field coupling to the spin of the electron.

Strictly speaking, the models with spin-orbit coupling do not have a quantized spin Hall conductance: .

Those models are more properly referred as topological insulator which is an example of topologically ordered states.

Topological Insulator is a material that behaves as an insulator in its interior while permitting the movement of charges on its boundary. In the quantum Hall effect, this effect occurs when there is a perpendicular magnetic field. There is a new type of topological insulator in which this occurs even when there is zero magnetic field. This is due to the Quantum spin Hall effect. It's called a topological insulator because its states are characterized by an index similar to the genus in topology, and it is an example of topologically ordered states.

Topological Order is a new kind of order (a new kind of organization of particles) in a quantum state that is beyond the Landau symmetry-breaking description. It cannot be described by local order parameters and long range correlations. However, topological orders can be described by a new set of quantum numbers, such as ground state degeneracy, quasiparticle fractional statistics, edge states, topological entropy, etc. Roughly speaking, topological order is a pattern of long-range quantum entanglement in quantum states. States with different topological orders can change into each other only through a phase transition.

In HgTe Quantum Wells

Real experimental systems, however are far from the idealized picture presented above in which spin-up and spin-down electrons are not coupled. A very important achievement was the realization that the quantum spin Hall state is robust to the introduction of spin-up spin-down scattering. In a separate paper, Kane and Mele introduced a topological Z₂ invariant who characterizes a state as exhibiting or not exhibiting a quantum spin Hall effect. Further stability studies of the edge liquid through which conduction takes place in the quantum spin Hall state proved, both analytically and numerically that the state is robust to both interactions and extra spin-orbit coupling terms that mix spin-up and spin-down electrons.

Since graphene has extremely weak spin-orbit coupling, it is very unlikely to support a quantum spin Hall state at temperatures achievable with today's technologies. A very realistic theoretical proposal for the existence of the quantum spin Hall state has been put forward by Bernevig, Hughes and Zhang^1: (BHZ) in Cadmium Telluride/Mercury Telluride/Cadmium Telluride (CdTe/HgTe/CdTe) quantum wells in which a thin (5-7 nanometers) sheet of HgTe is sandwiched between two sheets of CdTe. Different quantum wells of varying HgTe thickness can be built. When the sheet of HgTe in between the CdTe is thin, the system behaves like an ordinary insulator and does not conduct when the Fermi level resides in the band-gap. When the sheet of HgTe is varied and made thicker (this requires the fabrication of separate quantum wells), an interesting phenomenon happens. Due to the inverted band structure of HgTe, BHZ predicted that, at some critical HgTe thickness, a Lifshitz transition occurs in which the system closes the bulk band gap to become a semi-metal, and then re-opens it to become a quantum spin Hall insulator.

In the gap closing and re-opening process, two edge states are brought out from the bulk and cross the bulk-gap. As such, when the Fermi level resides in the bulk gap, the conduction is dominated by the edge channels that cross the gap. The two-terminal conductance is in the quantum spin Hall state and zero in the normal insulating state. As the conduction is dominated by the edge channels, the value of the conductance should be insensitive to how wide the sample is. A magnetic field should destroy the quantum spin Hall state by breaking time-reversal invariance and allowing spin-up spin-down electron scattering processes at the edge. All these predictions have been experimentally verified in an experiment performed in the Molenkamp labs at Universitat Würzburg in Germany.

Quantum Spin Hall Effect Glimpsed in HgTe

Physicists in Germany and the US have seen the first hints of an unusual solid-state phenomenon known as the quantum spin Hall effect (QSHE), in which spin-polarized electrons at the edges of an insulator are able to conduct. The researchers have seen this edge conduction in thin sheets of mercury telluride although they were not actually able to confirm that these edge electrons were spin-polarized. QSHE is interesting because it could be useful for making spintronic devices that exploit both the spin and charge of the electron.

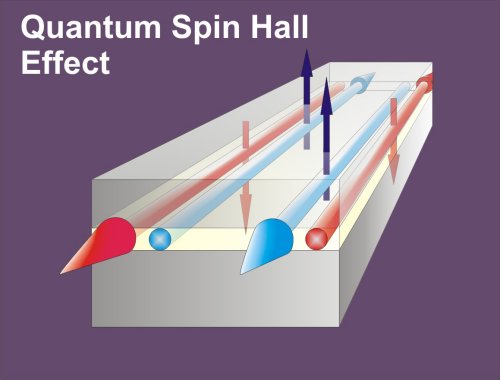
                                              Spins on The Edge                                                  

All physicists are familiar with the classic Hall effect, in which electrons moving in a thin sample in the presence of a transverse magnetic field experience a force that pushes them towards one side of the sample. This creates a charge imbalance that leads to a voltage across the material. In 2004, however, physicists obtained the first evidence for the "spin Hall effect", in which "spin-up" and "spin-down" electrons are deflected to opposite sides of a semiconductor. The resulting spin separation creates a spin current that is prependicular to the direction of the electric current.

The quantum spin Hall effect (QSHE) is a related phenomenon that has been predicted to occur not in conductors, but in certain very thin insulators. It involves spin-up electrons conducting along one edge of the insulator, with spin-down electrons conducting along the other side. Despite being insulators in the bulk, conduction is allowed at the edges because the interaction between the spin and orbital angular momentum of the electrons reduces the energy gap between the valence and conduction bands to zero for spin-polarized electrons.

Last year Shou-Cheng Zhang and colleagues at Stanford University in the US predicted that very thin sheets of mercury telluride (HgTe) should have the right band structure to support edge conduction. Now Zhang has joined forces with Laurens Molenkamp and colleagues at the University of Wuerzburg in Germany to find the first experimental evidence of QSHE in HgTe "quantum wells" -- sheets of material a few nanometers thick in which the electrons are confined to two dimensions.

Electrodes were attached to the wells to measure their conductance when cooled to about 30 mK. The same non-zero conductance predicted by Zhang was seen in all wells ranging in thickness from 6.3 to 12 nm. Because the conductance did not increase with well thickness, the researchers concluded that the conduction was occurring along the edges of the well, rather than in the bulk of the material.

However, wells thinner than 6.3 nm behaved like insulators – in agreement with Zhang's theory, which predicted that very thin wells would not have the appropriate band structure for the QSHE. When the team applied a magnetic field to the thicker wells, the edge conduction vanished – again in agreement with theory.

Despite their success at seeing edge conduction, the team was not able to confirm that the conducting electrons were spin polarized. "There is certainly more work to be done in this direction," says Charles Kane of the University of Pennsylvania, who has predicted that the QSHE should also occur in very thin sheets of carbon called graphene. "But I don't think that it detracts from the importance of this experiment", he added.

Molenkamp told physicsworld.com that the team is now exploring how a SQUID magnetic probe could be integrated within the quantum wells to measure the spin polarization of the edge electrons. And because spin-polarized edge electrons are expected to encounter no electrical resistance, edge-conducting insulators could be used in very low-power spintronic devices that use both the spin and charge of the electron to store and process information.

Fuente: http://en.wikipedia.org/wiki/Quantum_spin_Hall_effect, http://en.wikipedia.org/wiki/Topological_insulator, http://en.wikipedia.org/wiki/Topological_order, http://physicsworld.com/cws/article/news/31243
Nombre: Rodriguez B. Joiver I.
Asignatura: CRF

---

Get news, entertainment and everything you care about at Live.com. Check it out!